NCERT Solutions for Class 8 Maths Chapter 1 Rational Numbers Ex 1.1 are part of NCERT Solutions for Class 8 Maths. Here we have given NCERT Solutions for Class 8 Maths Chapter 1 Rational Numbers Ex 1.1.
| Board | CBSE |
| Textbook | NCERT |
| Class | Class 8 |
| Subject | Maths |
| Chapter | Chapter 1 |
| Chapter Name | Rational Numbers |
| Exercise | Ex 1.2 |
| Number of Questions Solved | 7 |
| Category | NCERT Solutions |
NCERT Solutions for Class 8 Maths Chapter 1 Rational Numbers Ex 1.2
Ex 1.2 Class 8 Maths Question 1.
Represent these numbers on the number line.
(i) \(\frac { 7 }{ 4 } \)
(ii) \(\frac { -5 }{ 6 } \)
Solution:
(i) For \(\frac { 7 }{ 4 } \), we make 7 markings of distance each on the right of zero and starting from 0. The seven marking is \(\frac { 7 }{ 4 } \).

The point P represents the rational number —.
(ii) For \(\frac { -5 }{ 6 } \), we make 5 markings of distance \(\frac { 1 }{ 6 } \) each on the left of zero and starting from 0. The fifth marking \(\frac { -5 }{ 6 } \) The point P represents the 6 rational number \(\frac { -5 }{ 6 } \).

Ex 1.2 Class 8 Maths Question 2.
Represent \(\frac { -2 }{ 11 } ,\frac { -5 }{ 11 } ,\frac { -9 }{ 11 } \) on the number line.
Solution:
To represent \(\frac { -2 }{ 11 } ,\frac { -5 }{ 11 } ,\frac { -9 }{ 11 } \) on a number line, draw a number line and mark a point O on it to represent zero. Now, mark a point P representing integers -1 on the left side of O on the number line.

Divide the segment OP into eleven equal parts. Let A, B, C, D, E, F, G, H, I, J be the points of division so that OA=AB =BC = … = JP. By construction, OB is two-eleventh of OP so B represents \(\frac { -2 }{ 11 } \) OE is five-eleventh of OP so E represents \(\frac { -5 }{ 11 } \) and OI is nine-eleventh of OP so I represents \(\frac { -9 }{ 11 } \).
Ex 1.2 Class 8 Maths Question 3.
Write five rational numbers, which are smaller than 2.
Solution:
Five rational numbers less than 2 may be taken
\(1,\frac { 1 }{ 2 } ,0,-1,\frac { -1 }{ 2 } \)
(There can be many more such rational numbers.)
Ex 1.2 Class 8 Maths Question 4.
Find ten rational numbers between \(\frac { -2 }{ 5 } \) and \(\frac { 1 }{ 2 } \).
Solution:
Converting the given rational numbers with the same denominators
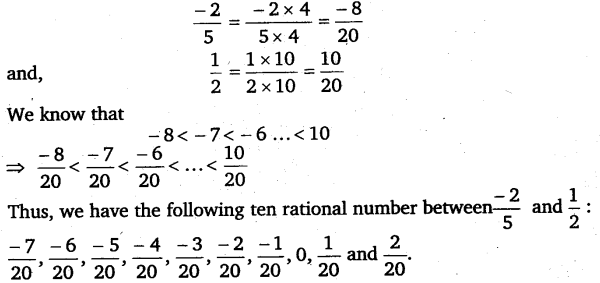
Ex 1.2 Class 8 Maths Question 5.
Find five rational numbers between

Solution:
(i) Converting the given rational numbers with the same denominators

(ii) Converting the given rational numbers with the same denominators


(iii) Converting the given rational numbers with the same denominators

Ex 1.2 Class 8 Maths Question 6.
Write five rational numbers greater than – 2.
Solution:
Five rational numbers greater than -2 may be taken as
\(-\frac { 3 }{ 2 } ,-1,\frac { -1 }{ 2 } ,0,\frac { 1 }{ 2 } \)
There can be many more such rational numbers.
Ex 1.2 Class 8 Maths Question 7.
Find ten rational numbers between \(\frac { 3 }{ 5 } \) and \(\frac { 3 }{ 4 } \).
Solution:
Converting the given rational numbers with the same denominators
