Reflection of Light From Plane Surfaces

Physics Topics can be challenging to grasp, but the rewards for understanding them are immense.

Reflection on a Plane Mirror

When a ray of light falls on a plane mirror (or any other plane surface), it is reflected according to some laws, called the laws of reflection of light. In order to understand the laws of reflection of light, we should first know the meaning of the terms : incident ray, point of incidence, reflected ray, normal (at the point of incidence), angle of incidence, and angle of reflection. So, we will discuss these terms first.

In Figure, we have a plane mirror MM’. The ray of light which falls on the mirror surface is called the incident ray. In Figure, AO is the incident ray of light. The incident ray gives the direction in which light falls on the mirror. The point at which the incident ray falls on the mirror is called the point of incidence.

In Figure, point O on the surface of the mirror is the point of incidence (because the incident ray AO touches the mirror surface at this point). We know that when a ray of light falls on a mirror, the mirror sends it back in another direction and we say that the mirror has reflected the ray of light.

The ray of light which is sent back by the mirror is called the reflected ray. In Figure, OB is the reflected ray of light. The reflected ray of light shows the direction in which the light goes after reflection from the mirror.

Another term that we will be using is the ‘normal’. The ‘normal’ is a line at right angle to the mirror surface at the point of incidence. In other words, ‘normal’ is a line which is perpendicular to the mirror at the point of incidence. In Figure 5, the mirror is MM’ and the point of incidence is O. So, the line ON is the normal to the mirror surface at point O.

The normal has been represented by a dotted line to distinguish it from the incident ray and the reflected ray. Please note that ‘normal’ is just a ‘perpendicular line’ to the mirror, and it should not be called the ‘normal ray’ like the incident ray or reflected ray.

The normal is a line at right angles to the mirror surface. In other words, normal makes an angle of 90° with the surface of the plane mirror. We will now discuss the angle of incidence and the angle of reflection.

The angle of incidence is the angle made by the incident ray with the normal at the point of incidence.
In other words, the angle between the incident ray and normal is called the angle of incidence. In Figure, the incident ray is AO and the normal is ON, so the angle AON is the angle of incidence. The angle of incidence is denoted by the letter i.

The angle of reflection is the angle made by the reflected ray with the normal at the point of incidence.
In other words, the angle between the reflected ray and normal is called the angle of reflection. In Figure, the reflected ray is OB and the normal is ON, so the angle NOB is the angle of reflection. The angle of reflection is denoted by the letter r. Keeping these points in mind, we will now describe the laws of reflection of light.

Laws of Reflection of Light

The reflection of light from a plane surface (like that of a plane mirror) or from a spherical surface (like that of a concave mirror or convex mirror) takes place according to two laws, which are known as the laws of reflection of light. The laws of reflection of light are given below :

1. First Law of Reflection. According to the first law of reflection of light: The incident ray, the reflected ray, and the normal (at the point of incidence), all lie in the same plane. For example, in Figure, the incident ray AO, the reflected ray OB and the normal ON, all lie in the same plane, the plane of paper. They are neither coming up out of the paper; nor going down into the paper.

2. Second Law of Reflection. According to the second law of reflection of light: The angle of reflection is always equal to the angle of incidence. We can also state the second law of reflection of light as follows by writing the angle of incidence first: The angle of incidence is equal to the angle of reflection. If the angle of incidence is i and the angle of reflection is r, then according to the second law of reflection :
∠i = ∠r
For example, if we measure the angle of reflection NOB in Figure, we will find that it is exactly equal to the angle of incidence AON.

The second law of reflection of light means that if the angle of incidence is 35°, then the angle of reflection will also be 35° (see Figure). And if the angle of incidence is changed to 45°, then the angle of reflection will also change and become 45° (see Figure). In every case, the angle of reflection remains equal to the angle of incidence.

We will now describe what happens when a ray of light falls normally (or perpendicularly) on the surface of a mirror. If a ray of light is incident normally on a mirror, it means that it is travelling along the normal to the mirror. So, the angle of incidence (i) for such a ray of light is zero (0). And since the angle of incidence is zero, therefore, according to the second law of reflection, its angle of reflection (r) will also be zero (0).

This means that a ray of light which is incident normally (or perpendicularly) on a mirror, is reflected back along the same path (because the angle of incidence as well as the angle of reflection for such a ray of light are zero). For example, in Figure 8, a ray of light falls on the plane mirror along the normal NO, therefore, it will be reflected along the same path ON.

So, in this case, the incident ray will be NO and the reflected ray will be ON. In other words, the same line represents incident ray, normal and reflected ray. We should, however, put two arrow heads on the same ‘normal’ line pointing in opposite directions – one arrow to represent incident ray and the other arrow to represent reflected ray (as shown in Figure).

Please note that the laws of reflection of light apply to all kinds of mirrors, plane mirrors as well as spherical mirrors (like concave mirrors and convex mirrors). By using the laws of reflection of light, we can find out the nature and position of the images (of objects) formed by the various types of mirrors.

Regular Reflection and Diffuse Reflection of Light

In regular reflection, a parallel beam of incident light is reflected as a parallel beam in one direction. In this case, parallel incident rays remain parallel even after reflection and go only in one direction (see Figure). Regular reflection of light occurs from smooth surfaces like that of a plane mirror (or highly polished metal surfaces).

For example, when a parallel beam of light falls on the smooth surface of a plane mirror, it is reflected as a parallel beam in only one direction as shown in Figure. Thus, a plane mirror produces regular reflection of light. Images are formed by regular reflection of light. For example, a smooth surface (like that of a plane mirror) produces a clear image of an object due to regular reflection of light.

A highly polished metal surface and a still water surface also produce regular reflection of light and form images. This is why we can see our face in a polished metal object as well as in the still water surface of a pond or lake. A polished wooden table is very smooth and hence produces regular reflection of light.

The regular reflection of light from a smooth surface can be explained as follows : All the particles of a smooth surface (like a plane mirror) are facing in one direction. Due to this the angle of incidence for all the parallel rays of light falling on a smooth surface is the same and hence the angle of reflection for all the rays of light is also the same. Since the angle of incidence and the angle of reflection are the same (or equal), a beam of parallel rays of light falling on a smooth surface is reflected as a beam of parallel light rays in one direction only (see Figure ).

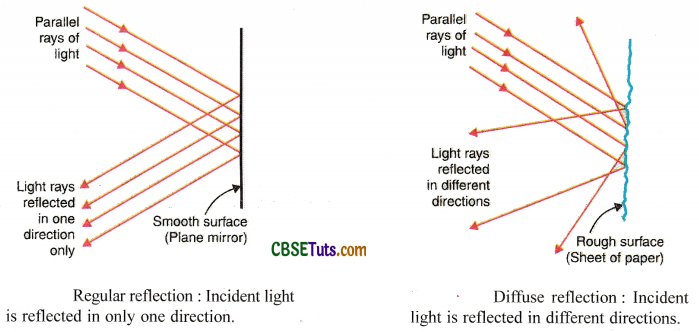
In diffuse reflection, a parallel beam of incident light is reflected in different directions. In this case, the parallel incident rays do not remain parallel after reflection, they are scattered in different directions (see Figure). The diffuse reflection is also known as irregular reflection or scattering.

The diffuse reflection of light takes place from rough surfaces like that of paper, cardboard, chalk, table, chair, walls and unpolished metal objects. For example, when a parallel beam of light rays falls on the rough surface of a sheet of paper, the light is scattered by making reflected rays in different directions (see Figure).

Thus, a sheet of paper produces diffuse reflection of light. No image is formed in diffuse reflection of light. For example, a rough surface (like that of paper) does not produce an image of the object due to diffuse reflection of light. Actually, the light rays falling on the rough surface of paper are scattered in all directions and hence no image is formed.

The diffuse reflection of light from a rough surface can be explained as follows : The particles of a rough surface (like that of paper) are all facing in different directions. Due to this, the angles of incidence for all the parallel rays of light falling on a rough surface are different and hence the angles of reflection for all the rays of light are also different.

Since the angles of incidence and the angles of reflection are different, the parallel rays of light falling on a rough surface go in different directions (see Figure). Please note that the diffuse reflection of light is not due to the failure of the laws of reflection. Diffuse reflection is caused by the roughness (or irregularities) in the reflecting surface of an object (like paper or cardboard, etc.). The laws of reflection are valid at each point even on the rough surface of an object.

The surfaces of most of the objects are rough (or uneven) to some extent. So, most of the objects around us cause diffuse reflection of light and scatter the light falling on them in all directions. In fact, we can see these objects only because they scatter light rays falling on them in all directions.

For example, a book lying on a table can be seen from all parts of the room due to diffuse reflection of light from its surface. The surface of book, being rough, scatters the incident light in all parts of a room. Hence the book can be seen from all parts of the room.

A cinema screen has a rough surface and causes diffuse reflection of light falling on it. The cinema screen receives light from a film projector and scatters it in all directions in the cinema hall so that people sitting anywhere in the hall can see the picture focused on the screen.

Objects and Images

In the study of light, the term ‘object’ has a special meaning. Anything which gives out light rays (either its own or reflected by it) is called an object. A bulb, a candle, a pin-head, an arrow, our face, or a tree, are all examples of objects from the point of view of study of light.

The objects can be of two types : very small objects (called point objects) or large objects (called extended objects). In drawing the ray-diagrams for the formation of images, we will be using both type of objects according to our convenience. In ray- diagrams, the point objects (like point sources of light or a pin-head) are represented by a ‘dot’, and the extended objects are represented by drawing ‘an arrow pointing upwards’.

In physics, image is an optical appearance produced when light rays coming from an object are reflected from a mirror (or refracted through a lens). This will become clear from the following example. When we look into a mirror, we see our face. What we see in the mirror is actually a ‘reflection’ of our face and it is called ‘image’ of our face.

Thus, when we look into a mirror, we see the image of our face in it. In this case, ‘our face’ is the ‘object’ and what we see in the mirror is the ‘image’. The image of our face appears to be situated behind the mirror. While watching a movie in the cinema hall, we see the images of actors and actresses on the cinema screen.

Please note that an image is formed when the light rays coming from an object meet (or appear to meet) at a point, after reflection from a mirror (or refraction through a lens). The images are of two types : real images and virtual images. These are discussed below.

Real Images and Virtual Images

The image which can be obtained on a screen is called a real image. In a cinema hall, we see the images of actors and actresses on the screen. So, the images formed on a cinema screen is an example of real images (see Figure 11)’. A real image is formed when light rays coming from an object actually meet at a point after reflection from a mirror (or refraction through a lens).

A real image can be formed on a screen because light rays actually pass through a real image. Real images can be formed by a concave mirror. A convex lens can also form real images. We will study the formation of real images with the help of ray- diagrams after a while.

The image which cannot be obtained on a screen is called a virtual image. A virtual image can be seen only by looking into a mirror (or a lens). The image of our face formed by a plane mirror cannot be obtained on a screen, it can be seen only by looking into the mirror. So, the image of our face in a plane mirror is an example of virtual image (see Figure).

Virtual images are also called unreal images. A virtual image is just an illusion. A virtual image is formed when light rays coming from an object only appear to meet at a point when produced backwards (but do not actually meet) after reflection from a mirror (or refraction through a lens). A virtual image cannot be formed on a screen because light rays do not actually pass through a virtual image.

A plane mirror always forms virtual images. Similarly, a convex mirror also forms only virtual images. A concave mirror can form a virtual image only when the object is kept within its focus. As we will study in the topic on lenses, a concave lens always forms virtual images but a convex lens forms a virtual image only when the object is within its focus. We will study the formation of virtual images with the help of ray-diagrams after a while.

We will now describe the formation of image in a plane mirror. Before we do that please remember that an object gives out a large number of light rays in all the directions but we will use only two light rays coming from the object to show the formation of image. This is done just to keep the ray-diagram simple.

Another point to be noted is that the real rays of light are represented by full lines (solid lines) whereas virtual rays of light are represented by dotted lines (broken lines). The real rays of light can exist only in front of a mirror. The virtual rays of light are those which we show behind a mirror.

The virtual light rays do not exist at all (because light cannot reach behind the mirror by passing through it). They just appear to be coming from behind the mirror. We will now describe how the image of an object is formed in a plane mirror by using the laws of reflection of light.

Formation of Image in a Plane Mirror

Consider a small object O (say, a point source of light) placed in front of a plane mirror MM’ (see Figure). The mirror will form an image I of the object O. Now, we want to know how this image has been formed. This happens as follows : The object O gives out light rays in all directions but we need only two rays of light to locate the image.

Now, a ray of light OA coming from the object O is incident on the plane mirror at point A and it gets reflected in the direction AX according to the laws of reflection of light making the angle of reflection r₁ equal to the angle of incidence’, (see Figure). Another ray of light OB coming from the object O strikes the mirror at point B and gets reflected in the direction BY, again making the angle of reflection r₂ equal to the angle of incidence i₂.

The two reflected rays AX and BY are diverging (moving away from one another), so they cannot meet on the left side. Let us produce the reflected rays AX and BY backwards (as shown by dotted lines in Figure). They meet at point I behind the mirror. Now, when the reflected rays AX and BY enter the Figure.

Formation of image in a plane mirror, eye of a person at position E, the eye sees the rays of light in the straight line direction in which the reflected rays enter it. So, the person looking into the mirror from position E sees the reflected rays as if they are coming from the point I behind the mirror (because I is the point of intersection of the reflected rays when produced backwards to the right side).

Thus, point I is the image of the object O formed by the plane mirror. For example, if our face is at position O in front of the plane mirror, then we will see the image of our face in the mirror at point I.

Please note that the image formed by a plane mirror can be seen only by looking into the mirror. So, if a screen is placed at position I, no image would be formed on it because the light rays do not actually pass through the point I, they only appear to do so.

An image of this type, which cannot be received on a screen, is known as a virtual image. Another point to be noted is that the light rays shown by dotted lines behind the mirror are only imaginary light rays. There can be no real light rays behind a mirror because it has a silvered reflecting surface at its back (covered with a paint) which does not allow light rays to pass through it and go behind the mirror.

So, there is no light coming to the person directly from the image point I. It is only the light reflected from the mirror which appears to be coming from the image point I.

Image of an Extended Object (or Finite Object)

We have just studied that a plane mirror forms a point image of a point object. But most of the actual objects are much bigger than a mere ‘point’ and they are called ‘extended objects’ or ‘finite objects’. An extended object (or bigger object) can be considered to be made up of a very, very large number of point objects.

So, the image of an extended object is a collection of the image points corresponding to the various points of the object. We will now describe how a plane mirror forms the image of an extended object. In our ray-diagrams, we will use an ‘arrow pointing upwards’ to represent an extended object (or finite object).

In Figure, an extended object AB (an arrow pointing upwards) is placed in front of a plane mirror MMIn order to locate the image of arrow AB in the plane mirror, we will first find out the positions of images of its top point A and bottom point B. This can be done as follows :

(i) From point A, we draw an incident ray AC perpendicular M to the mirror (see Figure). This will be reflected back along the same path. So, CA is the first reflected ray. We now draw another incident ray AD which strikes the mirror at point D. The ray AD is reflected along DE, making an angle of reflection (r₁) equal to the angle of incidence (i₁).

Thus, DE is the second reflected ray here. We produce the two reflected rays CA and DE backwards by dotted lines. They meet at point A’ (A-dash). So, A’ is the virtual image of the top point A of the object.

(ii) From point B, we draw an incident ray BE perpendicular to the mirror. This will be reflected back along the same path, giving the reflected ray FB. Another incident ray BG is reflected along GH making the angle of reflection (r₂) equal to the angle of incidence (i₂). We produce the two reflected rays FB and GH backwards by dotted lines. They meet at point B’ (B-dash). So, B’ is the virtual image of the bottom point B of the object.

In this way we have located the images of the top point A and bottom point B of the object. Now, each point of the object (or arrow) between A and B will give a point image which will lie between the points A’ and B’. So, to get the complete image of object AB, we join the points A’ and B’ by a dotted line. Thus, A’B’ (A-dash B-dash) is the complete image of the object AB which has been formed by the plane mirror (see Figure).

The image is virtual, erect (same side up as the object, because both the object and image have arrow-head at the top), and of the same size as the object. Please note that in Figure, the image A’B’ has been drawn by dotted line just to show that it is a virtual image.

Thus, the nature of image formed by a plane mirror is virtual and erect. And the size of image formed by a plane mirror is equal to that of the object. The image is at the same distance behind the plane mirror as the object (arrow) is in front of the mirror.

The Position of Image Formed in a Plane Mirror

The image formed in a plane mirror is at the same distance behind the mirror as the object is in front of the mirror (see Figure ). In other words, the image and object are at equal distances from a plane mirror but they are on the opposite sides of the plane mirror.

For example, if an object is placed at a distance of 5 cm in front of a plane mirror, then its image will also be formed at a distance of 5 cm behind the mirror. And the total distance between the object and its image will be 5 + 5 = 10 cm. That is, the object will be at a distance of 10 cm from its image.

Lateral Inversion

If we stand in front of a big plane mirror, we see the image of our body in it. Though our image appears to be just as we are, but there is a difference. This is because if we lift our right hand, then our image lifts its left hand. And if we lift our left hand, then the image appears to lift its right hand (see Figure).

This means that the right side of our body becomes left side in the image; whereas the left side of our body becomes right side in the image. It appears as if our image has been ‘reversed sideways’ with respect to our body. This effect of reversing the sides of an object and its image is called lateral inversion. And we say that the image formed in a plane mirror is laterally inverted. In other words, the image formed in a plane mirror is ‘sideways reversed’ with respect to the object. We can now define lateral inversion as follows :

When an object is placed in front of a plane mirror, then the right side of object appears to become the left side of image; and the left side of object appears to become the right side of image. This change of sides of an ‘object’ and its ‘mirror image’ is called lateral inversion.

The phenomenon of lateral inversion will become clear from the following example. Suppose we have a placard having the word RED written on it [see Figure (a)], When we hold this placard in front of a plane mirror, the image of word RED appears to be like ⱭƎЯ [see Figure (b)].

Please note that the object (placard) has the letter R on its left side but the image has this letter in reversed form Я on its right side. Similarly, the image of letter E appears to be reversed like Ǝ. And the letter D on the right side of the object (placard) is on the left side of the image in the reversed form as Ɑ.

Thus, all the letters written on the placard are reversed from left to right. We say that the image is laterally inverted. This is an example of lateral inversion. The phenomenon of lateral inversion is due to the reflection of light.

From the above discussion we conclude that the image formed in a plane mirror is laterally inverted (or sideways reversed) with respect to the object. It is due to lateral inversion that the image of our right hand appears to be our left hand.

So, when we sit in front of a plane mirror and write with our right hand, it appears in the mirror that we are writing with the left hand. And it is also due to lateral inversion that the parting in our hair on the right appears to be on the left when seen in a mirror. The word AMBULANCE on the hospital vans is written in the form of its mirror image as (see Figure).

This is because when we are driving our car and see the hospital van coming from behind in our rear-view mirror, then we will get the laterally inverted image of and read it as AMBULANCE. Since an ambulance carries seriously ill patients, we can make way for it to pass through and reach the hospital quicklv. We are now in a position to give all the characteristics of an image formed by a plane mirror.

1. The image formed m a plane mirror is virtual. It cannot be received on a AMBULANCE written in screen. the form of its mirror image.
2. The image formed in a plane mirror is erect. It is the same side up as the object.
3. The image in a plane mirror is of the same size as the object.
4. The image formed by a plane mirror is at the same distance behind the mirror as the object is in front of the mirror.
5. The image formed in a plane mirror is laterally inverted (or sideways reversed).

Uses of Plane Mirrors

1. Plane mirrors are used to see ourselves. The mirrors on our dressing table and in bathrooms are plane mirrors.
2. Plane mirrors are fixed on the inside walls of certain shops (like jewellery shops) to make them look bigger.
3. Plane mirrors are fitted at blind turns of some busy roads so that drivers can see the vehicles coming from the other side and prevent accidents.
4. Plane mirrors are used in making periscopes.

We will now solve some problems based on plane mirrors.

Example Problem 1.
An incident ray makes an angle of 35° with the surface of a plane mirror. What is the angle of reflection ?
Solution:
In order to find out the angle of reflection, we should first know the angle of incidence. In this case, the incident ray makes an angle of 35° with the surface of the mirror (see Figure), so the angle of incidence is not 35°. The angle of incidence is the angle between incident ray and normal. So, in this case, the angle of .incidence, will be 90° – 35°= 55°. Since the angle of incidence is 55 degrees, therefore, the angle of reflection is also 55 degrees. This is shown clearly in Figure.

Example Problem 2.
David is observing his image in a plane mirror. The distance between the mirror and his image is 4 m. If he moves 1 m towards the mirror, then the distance between David and his image will be :
(a) 3 m
(b) 5 m
(c) 6 m
(d) 8 m
Solution:
(i) In this problem, David is the object. Initially, the distance between the mirror and David’s image is 4 m. Since the distance of object from plane mirror is equal to distance of image from plane mirror, therefore, the distance of David (which is object), from the plane mirror is also 4 m (see Figure).

(ii) David moves 1 m towards the mirror. This means that the distance of David from mirror will be 4 – 1 = 3 m. Since the image is formed at the same distance behind the mirror as the object is in front of it, therefore, the distance of image from, mirror in this case will also be 3 m (see Figure)
Now, Distance of David from mirror = 3 m (in front)
And, Distance of image from mirror = 3 m (at the back)
So, Distance between David and his image = 3 m + 3 m
= 6 m
Thus, the correct option is : (c) 6 m.

Example Problem 3.
The rear view mirror of a car is a plane mirror. A driver is reversing his car at a speed of 2 m/s. The driver sees in his rear view mirror, the image of a truck parked behind his car. The speed at which the image of the truck appears to approach the driver will be :
(a) 1 m/s
(b) 2 m/s
(c) 4 m/s
(d) 8 m/s
Solution:
In a plane mirror, the object and its image always remain at the same distance from the mirror. So, when the car reverses at a speed of 2 m/s, then the image will also appear to move towards the mirror at the same speed of 2 m/s. So, the speed at which the image of truck appears to approach the car driver will be 2 m/s + 2 m/s = 4 m/s.

Thus, the correct option will be : (c) 4 m/s.