Contents
Physics Topics are often described using mathematical equations, making them precise and quantifiable.
Define Work and Factors on Which It Depends
We have already studied force and motion in the previous chapters of this book. We will now study that whenever a force makes a body move, then work is said to be done. For doing work, energy is required. When the work is done by human beings or animals (like horses), then the energy for doing work is supplied by the food which they eat.
And when the work is done by machines, then energy is supplied by fuels (such as petrol and diesel, etc.) or by electricity. When work is done, an equal amount of energy is used up. In this chapter we will study work, energy and power. Let us discuss the work first.
In ordinary language the word “work” means almost any physical or mental activity but in physics it has only one meaning: Work is done when a force produces motion.
For example, when an engine moves a train along a railway line, it is said to be doing work; a horse pulling the cart is also doing work; and a man climbing the stairs of a house is also doing work in moving himself against the force of gravity.

The work done by a force on a body depends on two factors :
- Magnitude of the force, and
- Distance through which the body moves (in the direction of force).
We can now define work as follows : Work done in moving a body is equal to the product of force exerted on the body and the distance moved by the body in the direction of force. That is,
Work = Force × Distance moved in the direction of force
But usually we write :
Work = Force × Distance
If a force F acts on a body and moves it a distance s in its own direction, then:
Work done = Force × Distance
W = F × s
This formula will be used to solve numerical problems on work. It should be noted that when a body is moved on the ground by applying force, then the work is done against friction (which opposes the motion of the body).

Please note that though most of the books use the term ‘distance’ in the definition of work but a few books also use the term ‘displacement’ in the definition of work. So, we can also write the definition of work as follows : Work done in moving a body is equal to the product of force and the displacement of the body in the direction of force. That is,
Work = Force × Displacement in the direction of force
or Work = Force × Displacement
or W = F × s
Thus, in the discussion on work, whether we use the term ‘distance’ or ‘displacement’, it will mean the same thing. We will now discuss the unit of work.
Unit of Work
Work is the product of force and distance. Now, unit of force is newton (N) and that of distance is metre (m), so the unit of work is newton metre which is written as Nm. This unit of work is called joule which can be defined as follows : When a force of 1 newton moves a body through a distance of 1 metre in its own direction, then the work done is known as 1 joule. That is,
1 joule = 1 newton × 1 metre
or 1 J = 1 Nm
Thus, the SI unit of work is joule which is denoted by the letter J. Work is a scalar quantity. It should be noted that the condition for a force to do work is that it should produce motion in an object. That is, it should make the object move through some distance.
If, however, the distance moved is zero, then the work done “on the object” is always zero. For example, a man may get completely exhausted in trying to push a stationary wall, but since there is no displacement (the wall not move), the work done by the man on the wall is zero (see Figure).

However, the work done on the body of the man himself is not zero. This is because when the man pushes the wall, his muscles are stretched and blood is displaced to the strained muscles more rapidly. These changes consume energy and the man feels tired.
Here is another example. A man standing still at a bus stop with heavy suitcases in his hands may get tired soon but he does no work in this situation. This is because the suitcases held by the man do not move at all. From the above discussion it is clear that it is not necessary that whenever a force is applied to an object, then work is done.
Work is done only when a force is able to move the object. If the object does not move on applying force, no work is done at all.
Work Done Against Gravity
The force of gravity of earth pulls everything towards the surface of earth. So, if we lift a book from a table, we do work against the force of gravity. Please note that when a body is lifted vertically upwards, then the force required to lift the body is equal to its weight.
So, whenever work is done against gravity, the amount of work done is equal to the product of weight of the body and the vertical distance through which the body is lifted.
Suppose a body of mass m is lifted vertically upwards through a distance h. In this case, the force required to lift the body will be equal to weight of the body,, m × g (where m is mass and g is acceleration due to gravity). Now,
Work done in lifting a body = Weight of body × Vertical distance
W = m × g × h
Where, W = work done
m = mass of the body
g = acceleration due to gravity
and h = height through which the body is lifted
We will use this formula to calculate the work done in all those cases where the object is being lifted upwards, against the force of gravity.

Above Figure shows a man of mass m climbing the stairs having a vertical height h. In this case the work done by the man in lifting his body upwards against the force of gravity of earth is m × g × h (where g is the acceleration due to gravity). We will now solve some numerical problems based on work.
Example Problem 1.
How much work is done by a force of 10 N in moving an object through a distance of 1 m in the direction of the force ?
Solution:
The work done is calculated by using the formula :
W = F × s
Here, Force, F = 10 N
And, Distance, s = 1 m
So, Work done, W = 10 × 1 J
= 10 J
Thus, the work done is 10 joules.
Example Problem 2.
Calculate the work done in lifting 200 kg of water through a vertical height of 6 metres (Assume g = 10 m/s2).
Solution:
In this case work is being done against gravity in lifting water. Now, the formula for calculating the work done against gravity is :
W= m × g × h
Here, Mass of water, m = 200 kg
Thus, the force exerted by the brakes on the car is of 9000 newtons. The negative sign shows that it is a retarding force. 1
The work done by the brakes can be calculated by using the relation :
W = F × s
Here, Force, F = 9000 N
Distance, s = 50 m
So, Work done, W = 9000 × 50 J
= 450000 J
= 4.5 × 105 J
Thus, the work done by the brakes is 4.5 × 105 joules.
Work Done by a Force Acting Obliquely
So far we have considered only that case of work in which the body moves in the direction of the applied force. This, however, is not always so. In many cases, the movement of the body is at an angle to the direction of the applied force.
For example, when a child pulls a toy car with a string attached to it, the car moves horizontally on the ground, but the force applied by the child is along the string held in his hand making some/angle with the ground. This is shown in Figure, in which the toy car moves along the horizontal ground surface OX but the force is being applied along the string OA, the direction of force making an angle θ with the direction of motion.

Please note that in such cases we cannot use the formula W = F × s to calculate the work done because the distance moved, s, is not exactly in the direction of force applied.
In this case, the whole of force F is not being used in pulling the toy car, only its horizontal component along the ground is the effective force pulling the toy car. We will now derive a formula for the work done when the body moves at an angle theta, θ, to the direction of force.
Formula for Work Done When a Body Moves at an Angle to the Direction of Force
Suppose a body lying at point O is being pulled by a man (see Figure). Now, though the body is moving on the horizontal floor and reaches point X after covering a distance s, but the force F is being applied in the direction of the string OA, making an angle θ with the direction of motion of the body.
Please note that in this case all the force F is not utilised in pulling the body, only the horizontal component of force F is the effective force which is pulling the body along the ground. Thus, the work done in pulling the body will be equal to the product of horizontal component of the force and distance moved by body.

In this case the horizontal component of force F is F cos θ and the distance moved is s. Thus, work done :
W = F cos θ × s
where F = force applied
θ = angle between the direction of force and direction of motion
and s = distance moved
All the problems on work done by a force acting
obliquely can be solved by using the formula : F cos θ × s. The most important point to remember while applying this formula is that 0 is the angle between the direction of motion of body and the direction of force applied.
The calculation of work done when a body moves at an angle to the direction of applied force will become clear from the following example.
Example Problem.
A child pulls a toy car through a distance of 10 metres on a smooth, horizontal floor. The string held in child’s hand makes an angle of 60° with the horizontal surface. If the force applied by the child be 5 N, calculate the work done by the child in pulling the toy car.
Solution:
We will first draw the diagram for this problem. The toy car is moving along horizontal floor, so we draw a horizontal line OX to show the direction of motion of the toy car (see Figure). Now, the force of 5 N is applied along the string tied to the toy car making an angle of 69° with the floor.
So, we draw another line OA making an angle of 60° with horizontal floor OX. In Figure, OX represents the direction of motion and OA represents the direction of force, the angle between them being 60°.

Now, we know that the formula for work done when a body moves at an angle to the direction of force is :
W = F cos θ × s
Here, Force, F = 5N
Angle, θ = 69°
And, Distance, s = 10 m
So, putting these values in the above formula, we get:
Work done, W = 5 × cos 60° × 10
Now, if we look up the table of natural cosines, we will find that cos 60° = 0.5. So, putting this value of cos 60° in the above relation, we get:
W = 5 × 0.5 × 10
= 25 J %
Thus, the work done is 25 joules.
Work Done When the Force Acts at Right Angles to the Direction of Motion
If the force acts at right angles to the direction of motion of a body, then the angle 9 between the direction of motion and direction of force is 99°. Now, cos 99° = 0, so the component of force, F cos 90°, acting in the direction of displacement becomes zero and hence the work done also becomes zero. That is,
Work done, W = F cos 90° × s
= F × 0 × s (Because cos 90° = 0)
Work done, W = 0
This means that when the displacement of the body is perpendicular (at 90°) to the direction of force, no work is done.
For example, if a man carries a suitcase strictly horizontally, he does no work with respect to gravity because the force of gravity acts vertically downwards and the angle between the displacement of the suitcase and the direction of force becomes 90°, and cos 90° becomes zero (Though the man carrying the suitcase horizontally may be doing work against the forces like friction and air resistance).

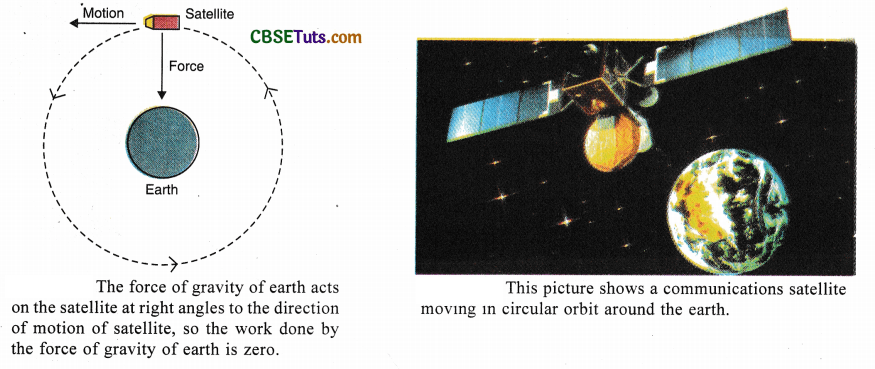
To keep a body moving in a circle, there must be a force acting on it directed towards the centre. This force is called centripetal 1 – 1 force. Now, the work done on a body moving in a circular path is also zero. This is because when a body moves in a circular path, then the centripetal force acts along the radius of the circle, and it is at right angles to the motion of the body.
Thus, the work done in the case of earth moving round the sun is zero, and the work done in the case of a Figure 13. A man carrying satellite moving round the earth is also zero. From this discussion it is clear that suitcases strictly horizontally it is possible that a force is acting on a body but still the work done is zero.
Work Done When the Force Acts Opposite to the Direction of Motion
If the force acts opposite to the direction of motion of a body, then the angle 0 between the direction of motion and the direction of force is 180°. In this case, the component of force F acting in the direction of motion of the body becomes, -F (minus F). So, the work done by the force is :
W = – F × s
It is obvious that the work done by the force in this case is negative. This means that when a force acts opposite to the direction in which the body moves, then the work done by the force is negative.
Positive, Negative and Zero Work
The work done by a force can be positive, negative or zero.
- Work done is positive when a force acts in the direction of motion of the body.
- Work done is negative when a force acts opposite to the direction of motion of the body.
- Work done is zero when a force acts at right angles to the direction of motion of the body.
Positive work done by a force increases the speed of a body : negative work done by a force decreases the speed of a body ; whereas zero work done by a force has no effect on the speed of a body. We will now give examples of positive work, negative work and zero work.
If we kick a football lying on the ground, then the football starts moving. The force of our kick has moved the football. Here we have applied the force in the direction of motion of football (see Figure). So, the work done on the football in this case is positive (and it increases the speed of football).
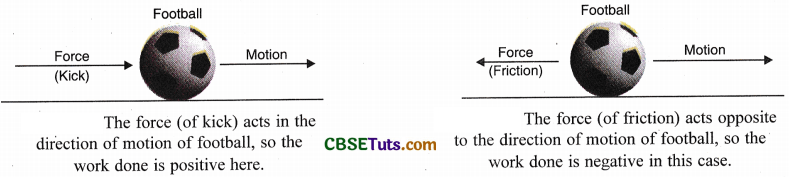
A football moving on the ground slows down gradually and ultimately stops. This is because a force due to friction (of ground) acts on the football. The force of friction acts in a direction opposite to the direction of motion of football (see Figure). So, in this case the work done by the force of friction on the football is negative (and it decreases the speed of football).
The satellites (like the moon) move around the earth in a circular path. In this case the gravitational force of earth acts on the satellite at right angles to the direction of motion of satellite (see Figure). So, the work done by the earth on the satellite moving around it in circular path is zero. Similarly, the work done by the sun on planets (like the earth) moving around it in circular orbits is zero.
When a boy throws a ball vertically upwards, then the force applied by the boy on the ball does positive work (because the force acts in the direction of motion of ball). But the gravitational force of earth acting on the upward going ball does negative work (because it acts opposite to the direction of motion of ball).